Rigidity of functors on spaces

8 Oct 2015 NUS mathematicians have recently discovered the homotopy rigidity of the functor ΣΩ on finite p-local co-H-spaces.

Rigidity is a common theme in mathematics under which a complete information of a collection of the objects under investigation can be detected by some information of the corresponding objects through certain constructive operations. The type of operations they consider is given in terms of functors. The based-loop functor Ω and the suspension functor Σ are fundamental operations in algebraic topology. Their main result is that both functors Ω and Σ are homotopy rigid on simply-connected p-local finite co-H-spaces. The result is obtained by a subtle interplay of homotopy decomposition techniques, modular representation theory and the counting principle.

The Steenrod problem of realizing polynomial cohomology rings initiatedthe cohomology rigidity problem in topology. This problem can be generalized by concerning the rigidity of functors. From the categorical view, the result of Bruner, Cohen and McGibbon can be restated as that the stabilization functor Ω^∞Σ^∞ is homotopy rigid on finite CW-complexes.A team led by Prof WU Jie from the Department of Mathematics in NUS reported that an unstable analogue of the result of Bruner, Cohen and McGibbon, where the methodology of modular representation theory plays an important role for solving the question.

The homotopy rigidity of the based-loop functor is a difficult problem with great importance in geometry, topology and physics. Under current technology, there are no results on the homotopy rigidity of the based-loop functor Ω that are derived directly by studying Ω. On the other hand, people know that many properties of co-H-spaces have the analogue for H-spaces through the duality of the notions, and the functors Ω and Σ are adjoint. Their research suggests a conjecture that the functor ΩΣ is homotopy rigid on simply-connected p-local finite H-spaces.

Nowadays cohomology rigidity is a common problem and investigates on which subcategory of topological spaces the homotopy type of a space is uniquely determined by its cohomology ring. Currently an important problem in toric topology is the cohomology rigidity for quasitoric.The interplay between homotopy theory and toric topology pushes forward the development of toric homotopy theory as one of the new directions in homotopy theory, where the closed connections between polyhedron products, Whitehead products and Hopf invariants provide a fundamental clue for developing toric homotopy theory.

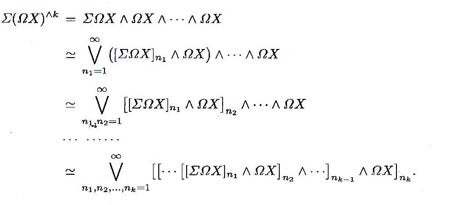
Decompositions of suspended self-smashes of loops on co-H-spaces. [Image credit: Wu Jie]    

References

1. Berstein I. “On co-groups in the category of graded algebras.” Trans. Amer. Math. Soc. 115 (1965) 257.

2. Buchstaber V, Grbić J. “Hopf algebras and homology of loop suspension spaces.” Topology, Geometry, Integrable Systems, and Mathematical Physics: Novikov’s Seminar 2012-2014, American Mathematical Society Translations Series 2, Advances in the Mathematical Sciences (2014) 75.

3. Grbić J, Theriault S, Wu J. “Suspension splittings and James-Hopf invariants.” Proc. Roy. Soc. Edinburgh Sect. A 144 (2014) 87.

4. Masuda M. “Cohomological non-rigidity of generalized real Bott manifolds of height 2.” Tr. Mat. Inst. Steklova 268 (2010) 252.